java File类的相关操作
本文共 527 字,大约阅读时间需要 1 分钟。
1.File类概述和构造方法
windows系统
- 一个点表示当前目录 直接写文件名字也是如此
- 两个点表示上级目录

注意第三个构造方法 父为对象 子为路径名

直接输出File对象会输出文件的绝对路径因为File类改写了toString()方法,输出时会自动调用
这三个构造方法做了同样的事情
2.File类的创建方法

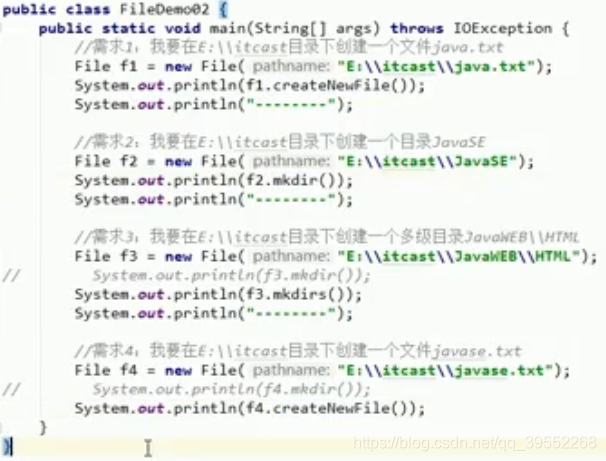
f4.mkdir()会创建一个名字为javase.txt的文件 如果这时再调用 f4.createNewFile会报false因为已经存在一个名字为javase.txt的目录,在这里目录就是文件的意思 mkdirs只能创建多层目录,如果想创建多层目录+txt文件就用mkdirs+creatNewFile

3.绝对路径和相对路径

4.delete和实例
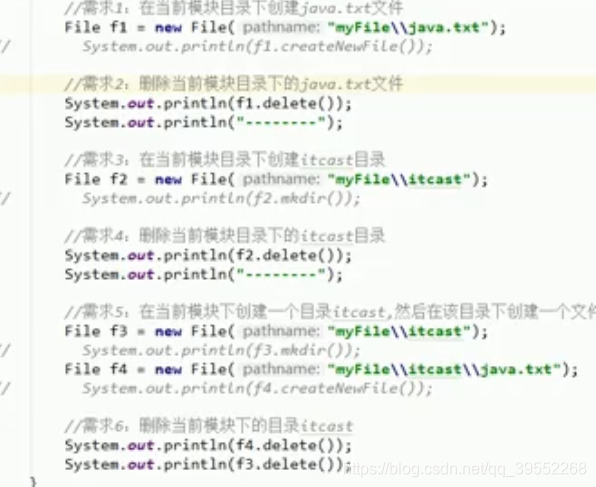
如果一个文件下有内容,则不能直接删除此文件
5.File常用方法
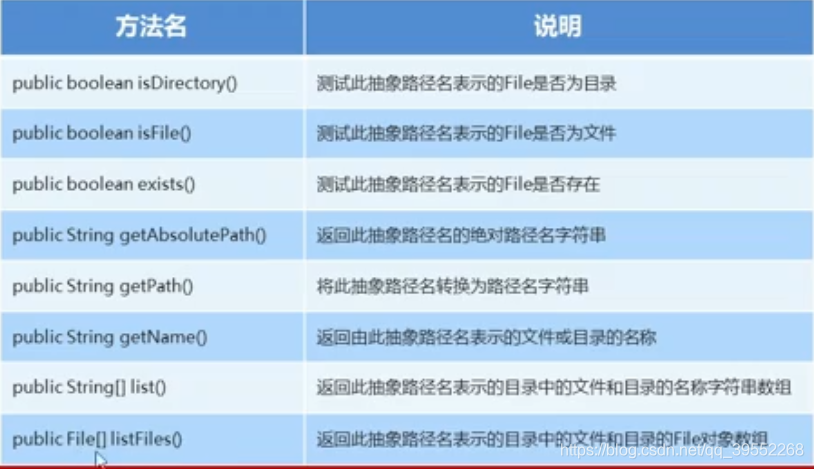 、
、
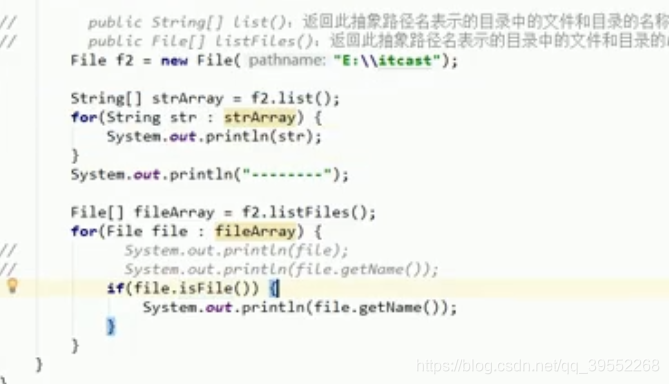
//抽象路径名 绝对路径名和 规范路径名的区别
file.list获取当前文件下的目录或文件的名字
就等于f2.listFiles()方法获取当前目录下所有文件和目录对象后在分别调用他们的getName()方法,但这个方法的好处就是可以通过文件对象来进行各种额外的判断操作如上面展示的
递归遍历一个目录下的所有文件

转载地址:http://ymsi.baihongyu.com/
你可能感兴趣的文章
mysql 为某个字段的值加前缀、去掉前缀
查看>>
mysql 主从
查看>>
mysql 主从 lock_mysql 主从同步权限mysql 行锁的实现
查看>>
mysql 主从互备份_mysql互为主从实战设置详解及自动化备份(Centos7.2)
查看>>
mysql 主从关系切换
查看>>
MYSQL 主从同步文档的大坑
查看>>
mysql 主键重复则覆盖_数据库主键不能重复
查看>>
Mysql 事务知识点与优化建议
查看>>
Mysql 优化 or
查看>>
mysql 优化器 key_mysql – 选择*和查询优化器
查看>>
MySQL 优化:Explain 执行计划详解
查看>>
Mysql 会导致锁表的语法
查看>>
mysql 使用sql文件恢复数据库
查看>>
mysql 修改默认字符集为utf8
查看>>
Mysql 共享锁
查看>>
MySQL 内核深度优化
查看>>
mysql 内连接、自然连接、外连接的区别
查看>>
mysql 写入慢优化
查看>>
mysql 分组统计SQL语句
查看>>
Mysql 分页
查看>>